How Do You Solve The Quadratic Using The Quadratic Formula
Section 4.6 The Discriminant The discriminant of a quadratic equation is the expression $$b^2-4ac$$ that appears under the square root in the Quadratic Formula.Click here 👆 to get an answer to your question ️ The discriminant of the quadratic equation 2x2 - 4x + 3 = 0 is(A) -8(B) 10(C) 8(D) 2/2Use the Zero Product Property to solve the following quadratic equation. (x - 7)(x + 2) = 0, Solve the following quadratic equation by factoring. x2+3x+2 = 0, Solve the following quadratic equation by factoring. x2 - 6x + 5 = 0, Solve the following quadratic equation by factoring. x2 - 6x - 27 = 0Stack Exchange network consists of 176 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers.. Visit Stack ExchangeQuadratic equations are the equations where polynomial has the degree two. Quadratic equations are the equations of type ax 2 + bx + c = 0 where x is unknown and a, b, c are known real numbers and a should not be zero. If a=0 then the equation will not remain quadratic, it will be then linear as a=0 will eliminate x 2 term. As the quadratic equation has the highest degree two, so this equation
The discriminant of the quadratic equation 2x2 - 4x + 3
14.12.2019 Math Secondary School Find the discriminant of the quadratic equation 2x²-4x+3=0 1 See answer murugeshanbabu1971 is waiting for your help. Add your answer and earn points. misraanay misraanay Answer: 16- 24 = -8. Equation has no real root. Step-by-step explanation: D = b^2 - 4ac. New questions in Math. 8. 5/6 of a journey is 200Suppose that you have a function represented by #f(x) = Ax^2 + Bx + C#.. We can use the quadratic formula to find the zeroes of this function, by setting #f(x) = Ax^2 + Bx + C = 0#.. Technically we can also find complex roots for it, but typically one will be asked to work only with real roots.The discriminant of the quadratic equation ax^2+bx+c=0 is D=b^2-4ac If D>0, then the equation has two distinct real solutions if D=0, then the equation has exactly one real solution if D<0, then the equation has no real solutionThe Discriminant. The quadratic formula not only generates the solutions to a quadratic equation, but also tells us about the nature of the solutions. When we consider the discriminant, or the expression under the radical, [latex]{b}^{2}-4ac[/latex], it tells us whether the solutions are real numbers or complex numbers and how many solutions of each type to expect.

Solving Quadratic Equations
Solve the equation using the Quadratic Formula. Question 4. x 2 + 41 = −8x Answer: Question 5. −9x 2 = 30x + 25 Answer: Question 6. 5x − 7x 2 = 3x + 4 Answer: Find the discriminant of the quadratic equation and describe the number and type of solutions of the equation. Question 7. 4x 2 + 8x + 4 = 0 Answer: qm 8. \(\frac{1}{2}\)x 2 + x74. r2-3 = 13 75. 2s +8 = 24 76. 22t -6 = 12 77. 23u +5 = 8 78. (v -2)2 +4 = 13 79. 23(w +4) -4 = 44 80. Two times the square of five more than a number is seventy-two. Write an equation that models this situation. Solve the equation. Solving by Completing the Square Class Work Fill in the blank to complete the square. 81. 2a + 8a +__Q. What is the discriminant and how many solutions would this quadratic have?-4x 2 - 8x - 8 = -4This lesson has an important part in learning the sum and the product of the roots of a quadratic equation. Activity: Fill In Directions: Find the sum and product. Let's recall Addition and Multiplication of signed numbers. x 1 x 2 SUM PRODUCT Ex.: 3 4 (3+4) = 7 [(3)(4)] = 12-6 2 8 -3 5 4 -7 2 -4 -6equation: y = 4x2 + 10 Square Roots Method Quadratic Formula 11 What method would you use to solve the equation: y = x2 + 10x + 3 Square Roots Method Complete the Square 12 The discriminant is 24. How many and what type of solutions are there ? 2 Real 1 Real 13 The discriminant is -10. How many and what type of solutions are there ?
Learning Outcome Define the discriminant and use it to classify answers to quadratic equations
The (*2*)
The quadratic method not only generates the answers to a quadratic equation, but in addition tells us about the nature of the answers. When we imagine the discriminant, or the expression underneath the radical, [latex]b^2-4ac[/latex], it tells us whether or not the answers are real numbers or advanced numbers and what number of solutions of each and every type to be expecting.
Let us explore how the discriminant affects the analysis of [latex] \sqrtb^2-4ac[/latex] in the quadratic components and how it is helping to resolve the solution set.
If [latex]b^2-4ac>0[/latex], then the quantity underneath the radical will be a positive worth. You can always to find the square root of a favorable quantity, so evaluating the quadratic components will result in two actual answers (one via adding the certain sq. root and one by means of subtracting it). If [latex]b^2-4ac=0[/latex], then you'll be taking the square root of [latex]0[/latex], which is [latex]0[/latex]. Since adding and subtracting [latex]0[/latex] each give the same result, the "[latex]\pm[/latex]" portion of the method does now not matter. There will likely be one actual repeated solution. If [latex]b^2-4ac<0[/latex], then the quantity beneath the radical might be a detrimental worth. Since you can't in finding the sq. root of a destructive number the usage of real numbers, there are no real answers. However, you'll be able to use imaginary numbers. You will then have two complicated answers, one through including the imaginary square root and one by means of subtracting it.The desk below summarizes the dating between the price of the discriminant and the answers of a quadratic equation.
Value of (*2*) Results [latex]b^2-4ac=0[/latex] One repeated rational resolution [latex]b^2-4ac>0[/latex], easiest sq. Two rational solutions [latex]b^2-4ac>0[/latex], now not an excellent square Two irrational solutions [latex]b^2-4ac<0[/latex] Two complicated solutions A General Note: The (*2*)For [latex]ax^2+bx+c=0[/latex], the place [latex]a[/latex], [latex]b[/latex], and [latex]c[/latex] are actual numbers, the discriminant is the expression under the radical in the quadratic formulation: [latex]b^2-4ac[/latex]. It tells us whether or not the solutions are real numbers or complex numbers and how many answers of each type to be expecting.
ExampleUse the discriminant to search out the nature of the answers to the following quadratic equations:
[latex]x^2+4x+4=0[/latex] [latex]8x^2+14x+3=0[/latex] [latex]3x^2-5x - 2=0[/latex] [latex]3x^2-10x+15=0[/latex] Show (*8*)Calculate the discriminant [latex]b^2-4ac[/latex] for every equation and state the anticipated kind of answers.
[latex]x^2+4x+4=0[/latex] [latex] \ b^2-4ac=\left(4\proper)^2-4\left(1\right)\left(4\proper)=0[/latex] [latex]\textual contentThere might be one repeated rational solution.[/latex] [latex]8x^2+14x+3=0[/latex][latex] \ b^2-4ac=\left(14\right)^2-4\left(8\right)\left(3\proper)=100[/latex] [latex]\text100 is a great sq., so there will be two rational answers.[/latex] [latex]3x^2-5x - 2=0[/latex][latex] \ b^2-4ac=\left(-5\proper)^2-4\left(3\proper)\left(-2\right)=49[/latex] [latex]\textual content49 is a really perfect sq., so there can be two rational answers.[/latex] [latex]3x^2-10x+15=0[/latex][latex] \ b^2-4ac=\left(-10\proper)^2-4\left(3\right)\left(15\right)=-80[/latex] [latex]\textThere can be two complex answers.[/latex] ExampleUse the discriminant to resolve how many and what kind of answers the quadratic equation [latex]x^2-4x+10=0[/latex] has.
Show (*8*)Evaluate [latex]b^2-4ac[/latex]. First word that [latex]a=1,b=−4[/latex], and [latex]c=10[/latex].
[latex]\startarraylb^2-4ac=\left(-4\right)^2-4\left(1\proper)\left(10\right)=16-40=-24\finisharray[/latex]
The result is a adverse number. The discriminant is unfavourable, so the quadratic equation has two advanced solutions.
In the final example, we can draw a correlation between the number and sort of solutions to a quadratic equation and the graph of its corresponding function.
ExampleUse the following graphs of quadratic purposes to determine what number of and what type of solutions the corresponding quadratic equation [latex]f(x)=0[/latex] may have. Determine whether the discriminant will likely be greater than, lower than, or equivalent to 0 for each.
a.
b.
c.
Show (*8*)a. This quadratic function does now not touch or cross the x-axis; subsequently, the corresponding equation [latex]f(x)=0[/latex] can have complicated answers. This implies that [latex]b^2-4ac<0[/latex].
b. This quadratic serve as touches the x-axis exactly once which implies there is one repeated strategy to the equation [latex]f(x)=0[/latex]. We can then say that [latex]b^2-4ac=0[/latex].
c. In our ultimate graph, the quadratic serve as crosses the x-axis twice which tells us that there are two real number solutions to the equation [latex]f(x)=0[/latex], and subsequently [latex]b^2-4ac>0[/latex].
We can summarize our results as follows:
(*2*) Number and Type of Solutions (*24*) of Quadratic Function [latex]b^2-4ac<0[/latex] two complicated answers will not cross the x-axis [latex]b^2-4ac=0[/latex] one actual repeated resolution will touch x-axis once [latex]b^2-4ac>0[/latex] two actual answers will go x-axis two timesIn the following video, we display extra examples of find out how to use the discriminant to explain the sort of solutions of a quadratic equation.
[embedded content material]
Summary
The discriminant of the quadratic formulation is the amount below the radical, [latex] b^2-4ac[/latex]. It determines the quantity and the type of solutions that a quadratic equation has. If the discriminant is sure, there are [latex]2[/latex] actual answers. If it is [latex]0[/latex], there is [latex]1[/latex] actual repeated solution. If the discriminant is negative, there are [latex]2[/latex] complicated solutions (however no actual solutions).
The discriminant can also tell us about the conduct of the graph of a quadratic function.
3x2 - 4x = 0 Solving By Using Quadratic Formula - Brainly.ph

Determine The Number Of Real Solutions Each Quadratic

Y= X 2 + 4 X + 5 - Mathhomeworkanswers Q&A
5.4: The Quadratic Formula - Mathematics LibreTexts

First Quarter - Chapter 2 - Quadratic Equation

PPT - QUADRATIC FUNCTIONS AND INEQUALITIES PowerPoint

PPT - Quadratic Formula And The Discriminant PowerPoint
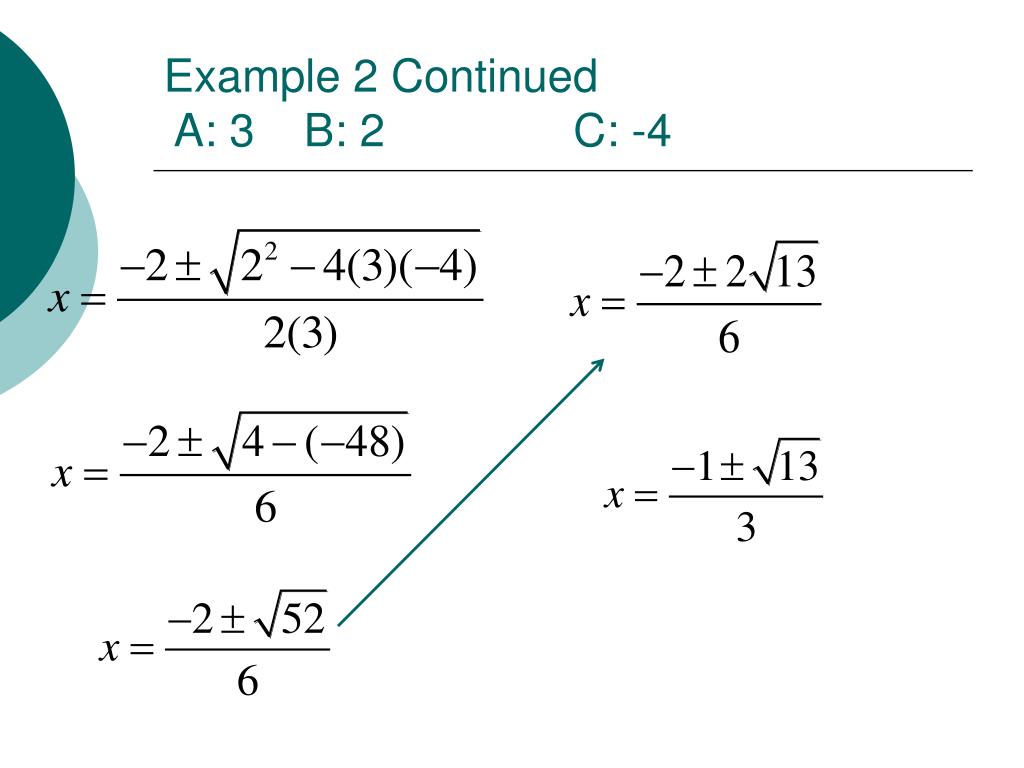
PPT - 5-3 PowerPoint Presentation, Free Download - ID:4954056

Determine The Nature Of The Roots Of The Following

Quadratic Functions By 1315026848

Rearrange The Quadratic Equation So That It Is Equal To 0
PPT - Solving Quadratic Equations By Graphing And

April 16, 2014

Pure Maths - Academic Skills Kit (ASK) - Newcastle University

SOLUTION: When Using The Quadratic Formula To Solve A
Determine The Discriminant For The Quadratic Equation –3

If [math]ad Ne Bc[/math], Then How Can You Prove That The
Solved: Es 1-4, Match The Quadratic Function With (11) Flx
R.D. Sharma Class 10 Solutions | Maths Chapter 8 Quadratic

Write The Discriminant Of The Quadratic Equation 2xsquare

Lecture 08 Quadratic Formula And Nature Of Roots

0 comments:
Post a Comment